PART 4.3 - Case study 3 - Corhampton 1988

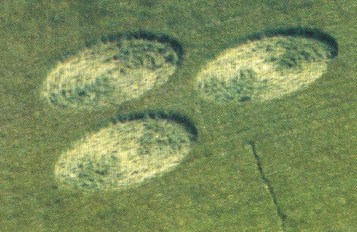
This formation is a so-called Triangular Triplet, which is to say, a grouping of three unconnected circles positioned such that they lie on the points of an equilateral triangle. Two such triplets appeared in 1988, one at Cheesefoot Head, the other at Corhampton:

Context: Billy Meier

This particular configuration has some history to it: The Andrews Catalogue mentions one appearing in North Yorkshire two years earlier, although it is unlikely D&D knew about it. But more importantly, triangular triplets not dissimilar to this one were reported and photographed in the Zurich Canton of Switzerland in the 1970s, and form part of the contentious Billy Meier accounts, which involved his claimed encounters with aliens. Meier photographed several triangular triplets in grass in the 1970s (I possess photos of six different ones), and although – for the record – I do not accept his stories, they do impact on the D&D saga, which is why I bring them up.

In 1987, the book Light Years (by Gary Kinder), appeared and told of Billy Meier’s experiences, but also included several remarks about these triplet formations, plus a photograph of one of them (above). Some extracts appeared in the UK press at the time. That the book appeared when it did, and included a photo remarkably similar to the two Triangular Triplets in crops seems more than chance. It is not certain which sections were extracted for the newspaper report D&D claim to have seen, but we do know that the book contains the image above plus the following text:

“…there was a meadow. There was very high grass, maybe a little less than a meter. And there were the six landing tracks... the grass was turned counter-clockwise… The tracks I looked at had stems bent over that weren’t broken and they never stood up."

At the public meeting in Marlborough in 1993, Doug Bower claimed to have created the two crop circle triplets of 1988 in direct response to this story. This is how Paul Fuller recorded it in Crop Watcher issue 18 (p5):

"The triangular triplets at Corhampton and the Cheesefoot Head punchbowl in 1988 were based on the triplet in Billy Meier's book "Light Years" which were publicised in an article in the September 13th 1987 News of the World colour magazine. Of course the earlier [crop circle] triplets were all three-in-a-line rather than in formation. D&D used a method similar to that used by the 'Dambusters' by using string attached to rods to get these three circles precisely positioned in an equilateral triangle. Because the rods bent as they pulled the string the Corhampton circles were less well positioned than the punchbowl circles."

And there, for D&D's supporters, the issue ends. D&D say they made it, and that's that.

However, I wish to delve a little deeper...

The Corhampton formation

 
In looking at the two triplet formations of 1988, both of which were (of course) included in Circular Evidence (Cheesefoot Head: p106-7; Corhampton: p108-111), I wish to focus on the second of them, the Corhampton triplet which was discovered on June 8, four days after the corresponding Cheesefoot Head circles. Corhampton, incidentally, lies in the countryside to the north of Portsmouth in Hampshire, and is therefore firmly in D&D’s territory. This is the better triplet to examine since the available evidence is superior – we have clear, near perpendicular aerial photographs and, unlike Cheesefoot Head, accurate measurements.

This formation was discovered and examined by the farmer, Charles Hall, in a field of barley. Hall contacted Colin Andrews that same day, and Andrews went over to inspect it. Andrews gives his measurements as follows, “There were three circles, each 10.8 meters (35.4 feet) in diameter… the circle edges were separated by 1.9 meters (6.2 feet) of standing crop (Circular Evidence p109).

According to the information gleaned from the Marlborough meeting, D&D used a method similar to that used by the Dambusters to get the circles on an equilateral grid. John Macnish adds a little in Crop Circle Apocalypse (p231), remarking: "Doug and Dave made the famous Corhampton triple circle formation using a technique Doug picked up from watching the film The Dambusters on TV."

So what is this technique? The Dambusters (RAF 617 Squadron), famously delivered the "bouncing bomb" during a World War II raid codenamed Operation Chastise. In order to have the bomb skim across water and crash into its target (a dam) they needed to ensure that the height of the plane was exactly right when the bomb was released. This they did by the use of trigonometry, shining two lights onto the water surface, each angled precisely so that when the plane was at the correct height, the two spots of light would be overlapping one another. Which is ingenious – but difficult to fathom in the context of crop circles.

Fuller's account states that they used string attached to rods, and that the rods bent while making the Corhampton formation, leaving it somewhat askew. But if Bower used rods and string, that has nothing to do with the Dambusters' technique. Three pieces of string of equal length simply need to be held taut to make an equilateral triangle. We might wonder how only two people could manage to make an equilateral triangle this way - surely it would need three people, one at each point holding their strings taut?

We might guess that the "rods" Bower mentioned were driven into the soil so as to act as this third person, although I am not aware that any holes were seen. There are other methods of course, but Bower has never explained exactly what he did. Nevertheless, when we look at the aerial photos, Bower seems to have a point. It does indeed look as if the formation is "askew", certainly not neatly aligned with the direction of the tramlines, anyway.

Revelation

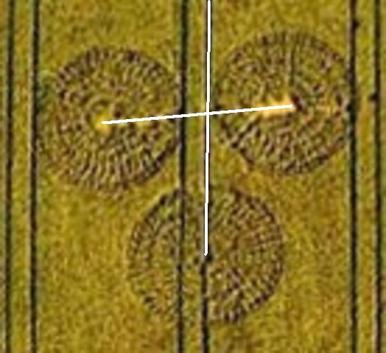
For Bower to say that the Corhampton triangle was less well positioned than the earlier formation, and claim that it was askew, betrays a comprehensive failure to understand the anatomy of the formation. Let's start our analysis by noting how the three circles are sized and situated such that if one draws tangent lines on their edges, they exactly fall in line with one another. A little closer together or a little further apart, or (using the same centre points for each circle) a little bigger or smaller, and this would not happen. To see it is a revelation, and it is undoubtedly a deliberate part of the design:

This detail is impressive enough, but here's the brilliant part: Notice what Bower took to be a lopsidedness in the formation - the way the upper two circles as we look are not precisely side-by-side. Here's why:

See that the lower circle in the photograph is centred on a tramline:

Note how the two circles above this are each sized and placed almost exactly between the adjacent sets of tramlines (there are minor inaccuracies, but this is clearly the intent).

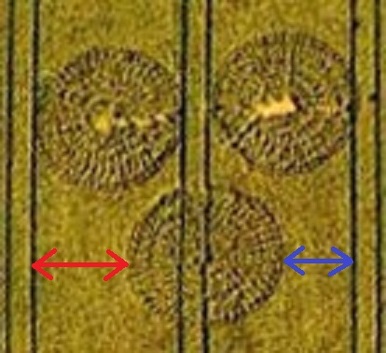
Now, note this: tramlines come in pairs, corresponding to pairs of tractor tyres. Because the lower circle is centred on one of the tramlines, and is therefore not precisely between the two which make up the pair, its outer edges are not equidistant from the next pairs of tramlines in the field. In other words, the circle is marginally closer to the set of tramlines to its right as we look, than to its left.

Since the formation uses the tramlines to define its boundaries, the only way that exact triangulation and tangential position can be achieved is if the other two circles are not side-by-side, and not aligned perpendicular to the tramlines. If they were, then the one on the right would be too close to the bottom circle, destroying the balance. To achieve the geometrical precision, the circle upper right needs to be positioned about 1 metre higher up in the photo.

And that's exactly what we are seeing. What Bower thought was lopsidedness is, when we look, the exact opposite, and shows that the positioning of all three circles, carefully pre-planned to achieve the implied tangent lines and equilateral geometry, with component circles snug within the boundaries created by the tramlines, was executed almost to perfection.

So what are we to think of Bower's claim to have made it, bizarrely invoking the Dambusters and claiming it was lopsided because of a bent rod? Can it really be the case that the geometry landed as it did in error?

Bower's diagram

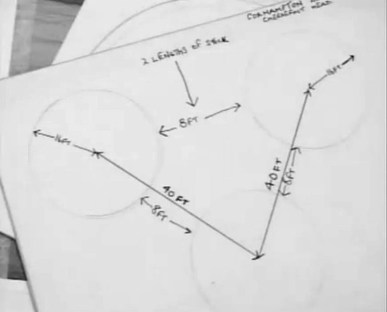
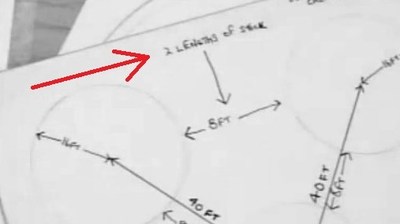
Let's remind ourselves of how Bower himself rendered this exquisite design on paper:

And since he must have known the formation's whole raison d'être was its exact equilateral symmetry, let's see how painstakingly he created perfect positioning, giving rise to tangent lines:

Besides amusing ourself with his apparent failure to understand the formation, there is something of significant interest in Bower's diagram: He includes measurements, which are worth checking through. According to the drawing, each circle is 16 feet in radius, conveniently (if ludicrously) four times the length of his stomper board. Moreover, the distance between the circles - the width of the standing crop between them - is given as 8 feet, or, as Doug says on his drawing, "2 lengths of stick", which is even more absurd (there is no reason why the gap should be a precise multiple of his stomper length).

So where do these figures come from, and do they correspond to the actual formation? Let's remind ourselves of Colin Andrews' measurements, obtained by site survey. In imperial, Andrews measured circle diameters of 35.4 feet, which is almost 18 feet radius, and a separation of 6.2 feet of standing crop between the circles. This is not what Bower says. Bower's drawing shows circle radii of 16 feet, about 2 feet smaller than actual, and the gap between them is 8 feet, 1.8 feet larger.

This might sound trivial, but the fact is, if we constructed the formation according to the dimensions given by Bower, the tangential layout would not even approximately occur. In other words, the dimensions given on his drawing would be unworkable when it came to actually constructing the formation, whose sizes were obviously very carefully thought through (and were not clumsy multiples of four).

I would like to prove this point, although I appreciate not all readers will be mathematically minded (so you can skip this bit if you prefer). I would like to apply some trigonometric principles to determine the spacing of three circles on an equilateral triangle, if they really were, as Bower says, 16 feet radius - to see if we get tangent lines.

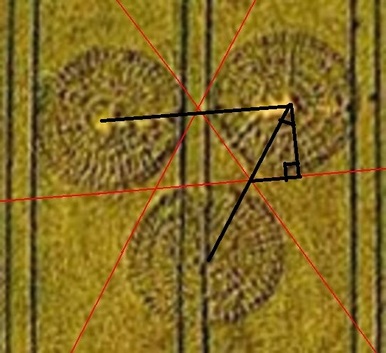
Let's start by constructing a right angled triangle in the formation, running from the centre of one of the circles to its perimeter - 16 feet long.

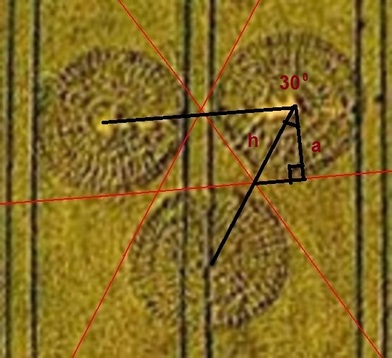
I have marked an angle at the top of the triangle, which must be 30 degrees, since the internal angle of the equilateral triangle is 60 degrees. Since we have constructed a right angled triangle, we can apply schoolboy trigonometry to calculate the missing dimensions. So, we label the triangle thus, where a is the adjacent side to the angle, and h is the hypotenuse:

Let's take Bower's radius (excuse the imperial), a as 16 feet. By using the formula h = a / cos angle, the hypotenuse will be 16 feet/cosine 30 = 16/0.87 = 18.4 feet. Note that the distance between any two circle centres will have to be exactly double this hypotenuse, because of the fact that they lie on tangents with one another:

So, we know that if the circle radii are 16 feet, then the circle centres will be spaced at two hypotenuse lengths, or 2 x 18.4 feet = 36.8 feet apart. To find out how far apart the circle edges will to be, in other words, how much standing crop there is between them, we simply subtract two radii from 36.8 feet, revealing the standing crop to be 36.8 - (2 x 16) = 4.8 feet wide.

Bower's "2 lengths of  stick" - 8 feet - is not merely inaccurate, it is almost double what is needed! Circles of 16 feet radius, separated by 8 feet of standing crop would not even approximately generate these tangents.

So how did Bower derive these impossible circle sizes? I have a theory: See that his diagram pertains to both the triangular triplets; both are named at the top. Now see Circular Evidence p107. Colin Andrews gives the diameters of the three circles in the earlier triplet at Cheesefoot Head, concluding that each circle is 10 metres diameter, or 5 metres radius. Converting to imperial, the radius comes out as 16 feet, 5 inches, and bingo! we have a match for Bower's own measurement of 16 feet. (Of course, he doesn't have the slightest idea how far apart they need to be nor that he needs to generate tangent lines, so he just uses 8 feet – two stompers.)

But let's check how Andrews obtained that dimension. He wasn't granted permission to enter the field and measure it, so: "To find the diameters I walked around the top of the hill to look along some faint tractor lines. I knew from the farmer that the lines in the field were 20 metres apart, and by marking their spacing on a piece of card 0.5 metres from my eye I had a scale to find their diameters, which proved to be 10 metres."

Note how crude this measurement technique is, and how the farmer gives an inch-perfect perfect 20 metres, from which Andrews derives a perfect 10. All of this has obviously been rounded off. For Bower to include on his drawing a radius accurate to Andrews' figures by a margin of just 5 inches is simply ridiculous.

There is no doubt that Bower's diagram does not show a blueprint for the construction of the acual formation, and the dimensions it gives are after-the-event estimates.

Details on the ground

Colin Andrews noted a very unusual effect on the floors of these circles: “About 35 per cent of the barley plants were compressed to the ground and swirled clockwise… The remaining plants had clearly also been flattened when the event occurred but had now lifted back to the sunlight in an extraordinary fashion… the plants had grouped themselves into oblong areas about 60 by 30 centimetres, and in three groupings the plant stems, along which there are three nodes or knuckles, were lifting up in a selective manner.” This effect had not been noted before, and is indicative that these circles were not made by the usual process.

Andrews continues, “I wanted to see this from the air, so I arranged a flight with Busty [Taylor] from Thruxton an hour or two later. We have experienced one surprise after another during our research, but few have matched the shock we had when we looked down on the Corhampton triple formation for the first time. The plants had now grown into a pattern consisting of seven concentric rings and forty-eight spokes. Each circle had the same form. We later found that plants inside an identical formation a few miles away [the Cheesefoot Head triplet] had formed the same dartboard or cartwheel pattern.”

The first thing to note is that to our knowledge, D&D’s circle-making methods had never caused this effect before. According to their own story, everything Colin Andrews had seen prior to 1987 was their handiwork, and yet Andrews had never encountered this effect. But we should note that circles are known with this effect afterwards, not least the Wiltshire giant circles of 1990, which D&D say they didn’t make (see The Latest Evidence p41 for a graphic example).

These circles, like the Corhampton triplet, were constructed extraordinarily accurately, to the extent that one, a three-ringed circle at Bishops Canning, was one day found to have acquired an extra ring around itself, more than 300 feet in diameter but only inches wide, and positioned so precisely that one could not perceive any eccentricity whatsoever. It was perfectly centred on the existing, very sizeable formation. Given the mathematical precision and unusual floor effect, my guess is that whoever made the Wiltshire circles of 1990 also made the meticulous Corhampton triplet of 1988. But so far as the Bishops Canning circle goes, we know for certain that it was not D&D, as they deny ever working in that area.

It is difficult to be precise about the floor details of the Corhampton triplet. Andrews counted seven concentric rings after a site visit, and I would broadly agree, although I find it difficult on the photographic evidence alone to be certain. But I think Andrews is likely to be there or thereabouts. I do believe that these effects are caused by some sort of stomper-board construction method in which concentric rings were pushed down, in a stepwise manner, repeatedly damaging the stems at the point of most impact. That is why we have these radial "spokes", which correspond to a stomper board being repeatedly forced down into the stems, as the hoaxer walks the rings.

The trouble is, Bower's stomper board was 4 feet long. To create a radius of about 18 feet, as here, Bower would have had to make his central circle first, then go around it four times. Yet there are certainly more rings visible in the floor lay than this. (This, incidentally, is not in dispute; at the London meeting in 1993, this very point was put to Bower, and Ken Brown suggested (on Bower's behalf) that the stomper might have overlapped adjacent lays.)

We could "do a Ken Brown" and create some explanation in our imaginations, supposing that each additional ring added considerably less than four feet to the diameter - but check these images which show Bower at work. Notice how little overlap there is between successive rings:

And think back to that drawing, above - Bower gives circle sizes as exact multiples of his four-foot stomper, indicating that it was designed to be constructed using a method which creates zero overlap on each ring. So did D&D create some special new technique just for this floor lay, to flatten out three simple, plain circles as Ken Brown guesses? I doubt it. To make an 18-feet radius circle, Bower's stomper board would only have created four rings in the floor lay, whereas there are about twice that number.

Conclusion

There is only one rational conclusion we can come to. Someone made this triplet, who was very careful in the design phase, incorporating perfect geometry and executing it to exacting standards. Circumstantial evidence indicates that they were inspired by the exposure of the earlier Billy Meier circles during 1987, but Bower - to judge by the hard evidence above - knew nothing about the implied tangents and positioning which make the formation such a success, thinking instead that the positioning of the circles was lopsided, and derived from multiples of his four foot stomper board.

It is only logical to conclude that the makers of this exceptionally fine geometrical layout were not D&D.



ON TO PART 5 - Supporters >